Branched Flow

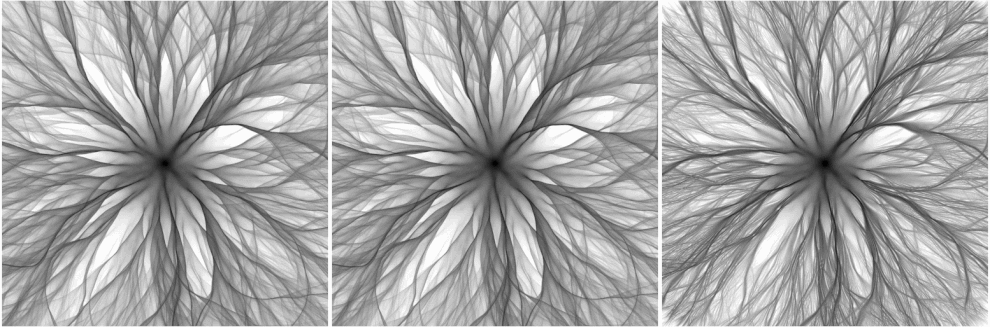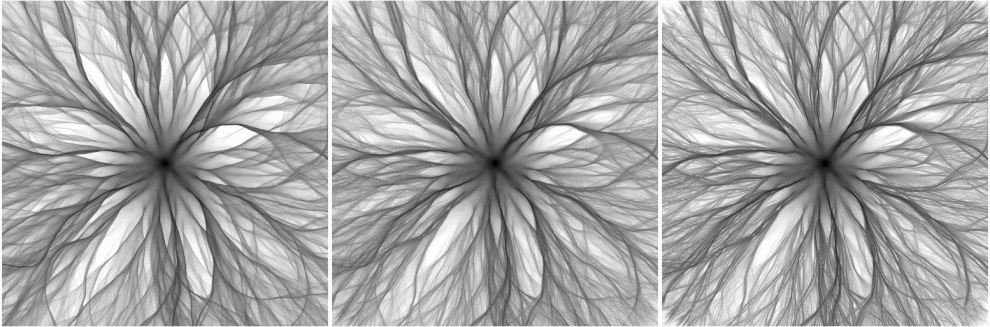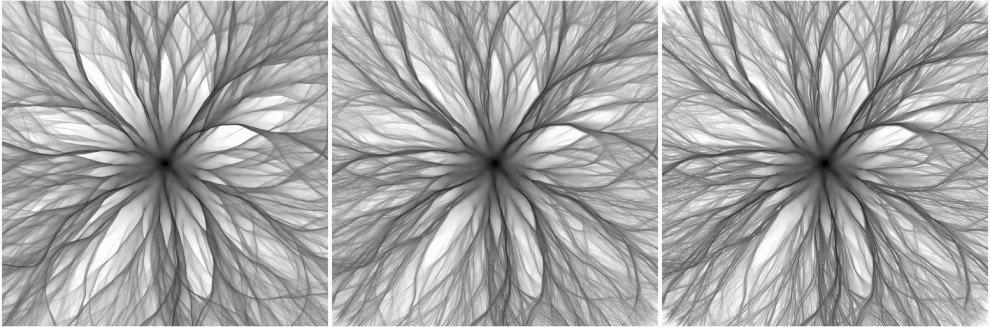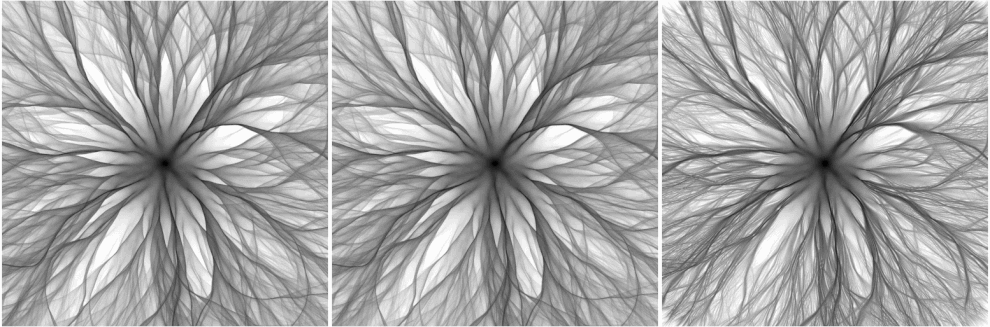
Branched flow refers to a phenomenon in wave dynamics that produces a tree-like pattern, involving successive mostly forward scattering events by smooth obstacles deflecting traveling rays or waves. This term was first coined in 2001 on a paper where the diffusion of electrons in 2D electron gas was studied. Professor Westervelt at Harvard found that instead of the expected diffusion fans, electrons flowed creating strong branches. These branches showed high intensity at long distances from the sources. Professor Eric J. Heller and others came to the conclusion that these branches were produced by the weakly refracting medium, that through successive kicks on the wave not only created focal points, but was able to keep the branches stable for distances much longer than the correlation length of the medium.

However, the first paper that we are aware of related to branched flow was in a rather different context. Wolfson and Tomsovic, at Washington State University, studied the propagation of sound through the ocean in 1999. They found that some bundles of trajectories evolving under the action of a random potential remained together for very long distances. Although the scales between the electron flow and the sound propagation were orders of magnitude apart, the underlying mechanism that give rise to both branched patterns is the same.

Branched flow is ubiquitous in nature: so far it has been reported from the micron scale in semiconductors to hundreds of kilometres in tsunami waves travelling through the ocean. But it is probably present even at smaller/larger scales: it is believed to be responsible for resistivity in the interaction between electron and phonons in metals, which takes place at the nanometre scale, and it is also been proposed to account for the large structure of the universe in relation with the Zeldovitch model. Actually this is not surprising, as often argued, every object in the physical world can be studied as a wave, and branched flow is a common behaviour of waves. Once you learn about it, you will start to discover branched flow everywhere, from water reflections of a pool to the sound of an airplane taking off.

However, branched flow has been unnoticed until recent years. It is arguably a regime as common as standard diffusion governed by Fick’s laws, and while diffusion appears in every textbook from undergraduate levels, hardly anyone knows about branched flow. There are several reasons that could explain it. First, branched flow is a transient regime. If you look sufficiently far away from the source, branched flow becomes regular diffusion. Nevertheless, quite often the transient regime is all you get. Tsunami waves hit the coastlines with all the power of the stable branches way before normal diffusion takes over. Physicists have many times neglected transient regimes because of their complicated mathematical nature, but in reality you get a lot of transient regimes (and not so much of the asymptotic). This complexity is precisely another reason for the long silence on branched flow. It requires the full power of a computer to appreciate and understand these intricate patterns. As happened with fractal geometry, that waited undercover until the 20^th century despite being visible in a plethora of facets of nature, branched flow has needed the right circumstances to be observed in its full splendour.

Despite of the wave origins of branched flow, it can be understood in terms of ray-tracing. By virtue of the eikonal approach, or using semiclassical methods, we can study branched flow using classical trajectories. Indeed, classical manifolds (sets of trajectories that resemble portions of the Euclidean space) are preferred. Due to the interaction of the waves or rays with the medium, these manifolds stretch and fold giving rise to fold catastrophes or caustics. These focal points accumulate a high density of trajectories and can persist for long times. Stable regions with high density in phase space are precisely what the eye perceives in real space as branches. Probably the simplest dynamical system capable of producing such behaviour is the so-called kick and drift map. The kick and drift map is describes in the following paragraph.

Hint: If you don't see controls to start a simulation. please scroll down a bit